ENERGY STORAGE SYSTEM

The battery has been one of the most commonly used sources of electric energy since the invention of the electric cell in 1800 by the Italian physicist Alessandro Volta ¹.

Components of a battery

A electrochemical battery is made up of one or more basic elements, which are grouped in series-parallel connections ².

An element is primarily composed of three components:

• Negative electrode or anode – the reducing electrode or donor – this donates electrons to the external circuit and where oxidation occurs during the electrochemical reaction;
• The positive electrode or the cathode – the oxidizing electrode – this accepts electrons from the external circuit and where the reduction process occurs during the electrochemical reaction;
• The electrolyte – the medium that conducts ions – is the medium in which energy transfer occurs, as ions, inside the element, between the anode and cathode. An electrolyte is usually a liquid such as water or other solvents, in which salts, acids, or alkalis are dissolved. There are also elements that have a solid electrolyte structure and exhibit ionic conduction at the operating temperature of the cell.

As anode and cathode, combinations of the lightest materials are used that provide the highest voltage and capacity for the cell. Such combinations are not always practical due to their reactivity with other elements of the cell, handling difficulties, high costs, etc.

In a practical system, the anode is chosen considering the following properties:

• To be efficient as a reducing agent;
• High yield [\(Ah/g\)];
• Stability;
• Ease of manufacturing;
• Low cost.

From the table below, it follows that hydrogen (\(H_2\)) is attractive as an anode material, but considering that it is difficult to handle and dangerous, it has not been widely used in practice. In practice, metals are the materials used for the anode, and for this purpose, zinc (\(Zn\)) has been predominantly used due to its suitable properties. Lithium (\(Li\)) is now more commonly used due to its low weight and high efficiency [\(Ah/g\)], and electrolytes have been developed that allow for its use with a certain degree of safety ².

Table 1. Characteristics of materials used for Anod²

Material	Molecular mass	Standard reduction potential at \(25^0C\)	Valence	Melting point	Density	Electrochemical equivalents
	[\(g\)]	[\(V\)]		[\(^0C\)]	[\(g/cm^3\)]	[\(Ah/g\)]	[\(g/Ah\)]	[\(Ah/cm^3\)]
\(H_2\)	2.01	0; -0.83*	2	-	-	26.59	0.037
\(Li\)	6.94	-3.01	1	180	0.54	3.86	0.259	2.06
\(Na\)	23.0	-2.71	1	98	0.97	1.16	0.858	1.14
\(Mg\)	24.3	-2.38; -2.68*	2	650	1.74	2.20	0.454	3.8
\(Al\)	26.9	-1,66	3	659	2.69	2.98	0.335	8.1
\(Ca\)	40.1	-2.84; -2.35*	2	851	1.54	1.34	0.748	2.06
\(Fe\)	55.8	-0.44; -0.88*	2	1528	7.85	0.96	1.04	7.5
\(Zn\)	65.4	-0.76; -1.25*	2	419	7.14	0.82	1.22	5.8
\(Cd\)	112.4	-0.40; -0.81*	2	321	8.65	0.48	2.10	4.1
\(Pb\)	207.2	0.13	2	327	11.34	0.26	3.87	2.9

Table 2. Characteristics of materials used for Cathode²

Material	Molecular mass	Standard reduction potential at \(25^0C\)	Valence	Melting point	Density	Electrochemical equivalents
	[\(g\)]	[\(V\)]		[\(^0C\)]	[\(g/cm^3\)]	[\(Ah/g\)]	[\(g/Ah\)]	[\(Ah/cm^3\)]
\(O_2\)	32.0	1.23; 0.40*	4	-	-	3.35	0.30
\(Cl_2\)	71.0	1.36	2	-	-	0.756	1.32
\(SO_2\)	64.0	-	1	-	-	0.419	2.38
\(MnO_2\)	86.9	1.28	1	-	5.0	0.308	3.24	1.54
\(NiOOH\)	91.7	0.49*	1	-	7.4	0.292	3.42	2.16
\(CuCl\)	99.0	0.14	1	-	3.5	0.270	3.69	0.95
\(FeS_2\)	119.9	-	4	-	-	0.89	1.12	4.35
\(AgO\)	123.8	0.57*	2	-	7.4	0.432	2.31	3.2
\(Br_2\)	159.8	1.07	2	-	-	0.335	2.98
\(HgO\)	216.6	0.10*	2	-	11.1	0.247	4.05	2.74
\(Ag_2O\)	231.7	0.35*	2	-	7.1	0.231	4.33	1.64
\(PbO_2\)	239.2	1.69	2	-	9.4	0.224	4.45	2.11
\(I_2\)	253.8	0.54	2	-	4.94	0.211	4.73	1.04

*Basic electrolyte, the rest of the data is for acid electrolyte

Classification of batteries

Electrochemical batteries are primarily divided into two categories²:

• Primary batteries, where the electrochemical process is irreversible, these being single-use, as was the electric cell of Alessandro Volta. These are generally encountered under the simple name of batteries;
• Secondary batteries, in which the electrochemical process is reversible, can be reused through recharging, first appearing in 1836, under the name of Daniell cell, which was invented by the British chemist and meteorologist John Frederic Daniell³. They are generally referred to as storage batteries or more simply as accumulators;
• Reserve batteries, in this type of battery the electrochemical process is not initiated as a result of isolating one of the three elements that make up the battery: the positive electrode (cathode), the negative electrode (anode), and the electrolyte. Usually, in this type of battery, the electrolyte is the component that is isolated, and materials such as water or seawater are used in the production of the electrolyte. They are used in applications where devices/equipment are in standby, and when necessary, this type of battery is activated and is capable of supplying high currents in a short time. They are used in the defense sector: in missiles, torpedoes, etc. ⁴.
  There are also storage batteries where direct current electricity is not transformed through electrochemical processes for the purpose of storage. In these storage batteries, direct current electricity is stored in the form of electric charge, as is the case with capacitor batteries. These began to be used with the technological development that allowed the creation of capacitors on the order of farads ⁵.

Operation of an element

The electrochemical processes during discharge and charging within a cell are presented in the figures below².

Figure 1. Cell discharge process	Figure 2. Cell charge process

Discharge of an element

In Figure 1, the discharge process is schematically presented. This process occurs when an element or a battery is connected to an external circuit, under an external load, during which electrons will move from the anode to the cathode through the external load. At the anode, the process of oxidation and electron donation will take place, which will be accepted by the cathode where the process of reduction will occur. Within the electrolyte, there will be a flow of anions (negative ions) towards the anode and a flow of cations (positive ions) towards the cathode².

From an electrochemical perspective, the discharge reaction can be written, assuming a metal as the anodic material, for example zinc (\(Zn\)) and a cathodic material, such as chlorine (\(Cl_2\)), as follows:
At the negative electrode, we have an anodic reaction (oxidation, loss of electrons):

\[ Zn \longrightarrow Zn ^{2+}+2e\]

At the positive electrode, we have a cathodic reaction (reduction, gain of electrons):

\[ Cl_2+2e \longrightarrow 2Cl^-\]

The overall reaction, in the case of discharge, is obtained by summing the two reactions at the anode and cathode, yielding:

\[ Zn + Cl_2 \longrightarrow Zn ^{2+} +2 Cl ^- (ZnCl_2) \]

Charging an element

In Figure 2, the charging process is schematically presented. During this process applied to a rechargeable element or secondary batteries, the direction of current flow is reversed, and as a result, oxidation will occur at the positive electrode and reduction at the negative electrode. Since the anode is, by definition, the electrode where oxidation occurs and the cathode is the one where reduction takes place, the positive electrode is now the anode and the negative one is the cathode ².

In the case of the element \(Zn – Cl_2\), previously described, the reaction during charging can be written as follows:

• At the negative electrode, we have a cathodic reaction (of reduction, of gaining electrons):

  \[ Zn^{2+}+2e \longrightarrow Zn\]

• At the positive electrode, we have an anod reaction (of oxidation, of electron loss):

\[ 2Cl^- \longrightarrow Cl_2+2e\]

The total reaction, in the case of charging, is obtained by summing the two reactions at the anode and respectively the cathode, yielding:

\[ Zn^{2+}+2Cl^- \longrightarrow Zn+Cl_2 \]

The voltage, capacity, and theoretical energy of an element

These three physical quantities: voltage, capacity, and energy, are primarily determined by the materials that make up the three components, namely the anode, cathode, and electrolyte, corresponding to an element. By combining the materials used for the anod, Table 1, and cathode, Table 2, batteries or battery elements with the following characteristics can be obtained.

Table 3. Voltage, capacity, and energy of primary batteries - Theoretical and practical values²

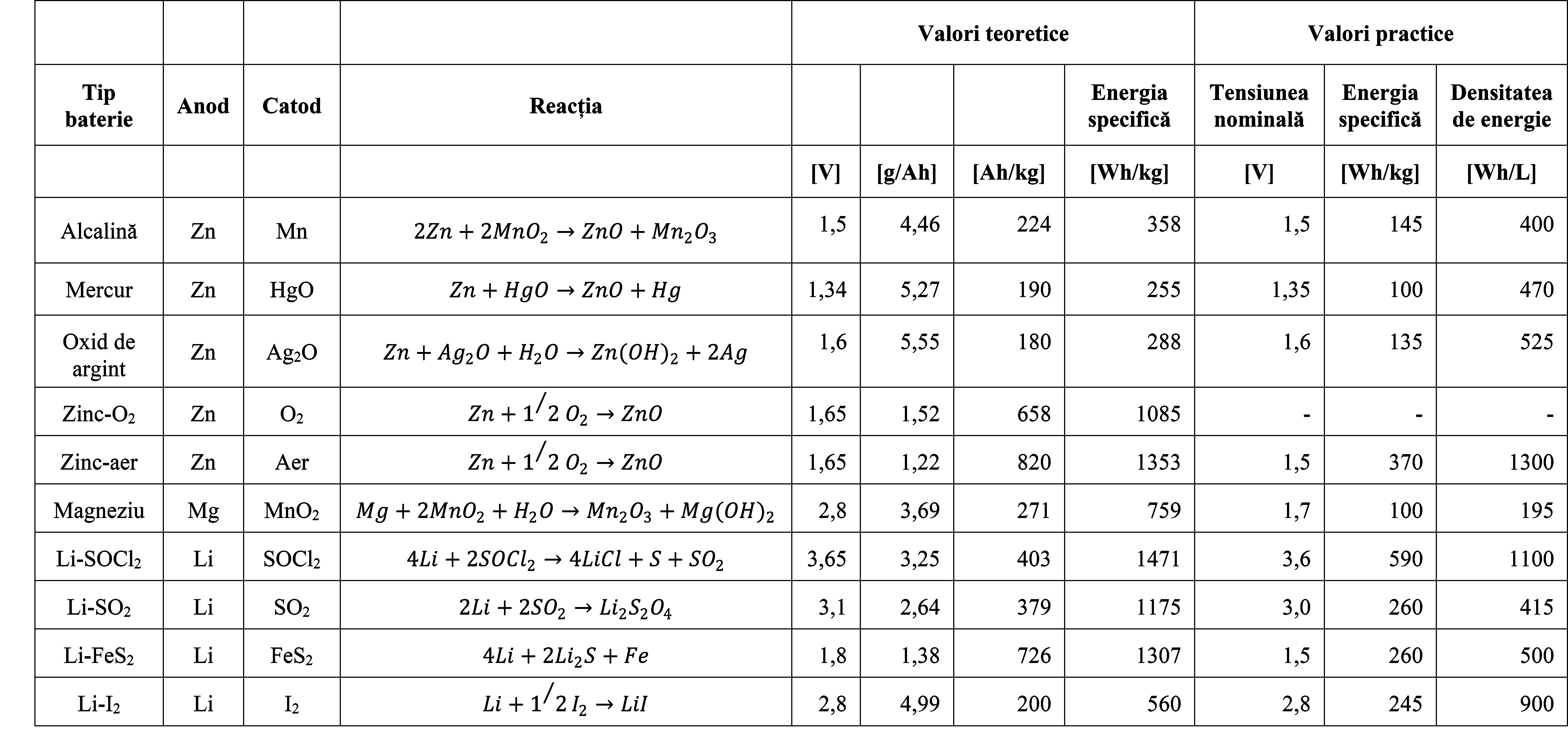
Table 4. Voltage, capacity, and energy of secondary batteries - Theoretical and practical values²

Free energy

Whenever a reaction occurs, the free energy of the system will be released, expressed as:

\[ \Delta G^0=-nFE^0\]

, where:
\(F\) – represents Faraday's constant (96,500 [C] or 26.8 [Ah]);
\(n\) – represents the number of electrons involved in the reaction;
\(E^0\) – represents the standard potential/voltage, in [V].

Theoretical voltage

This quantity of an element is determined based on the types of materials that make up the respective element. It can be determined from the free energy or can be obtained experimentally. Tables 1 and 2 indicate several materials that can be used for electrodes with the corresponding electrode potential/voltage².

The standard theoretical voltage of an element can be calculated from the standard potentials of the electrodes as follows:

Anode (oxidation voltage) + Cathode (reduction voltage) = Standard theoretical voltage

For example, in the case of the reaction:

\[ Zn+Cl_2 \longrightarrow ZnCl_2\]

the theoretical standard voltage will be obtained as follows:

\(Zn \longrightarrow Zn^{2+}+2e\)	\(-(-0.76 [V])\)
\(Cl_2 \longrightarrow 2Cl^--2e\)	\(1.36 [V]\)
.	\(E^0=2.12 [V]\)

Theoretical capacity

The theoretical capacity of an element is directly proportional to the amount of active material in the element that constitutes the electrodes. Capacity is defined in coulombs \([C]\) or in ampere-hours \([Ah]\). Most often, \([Ah]\) is used, where \(1 [Ah] = 3,600 [C]\). Theoretically, an equivalent weight of 1 gram of material will provide \(96.487 [C]\) or \(26.8 [Ah]\). The theoretical capacity is obtained from Faraday's law of electrolysis ⁶:

\[ q = {{z \cdot F} \over M} \]

, where:
\(q\) – represents the theoretical capacity, \([Ah/g]\);
\(z\) – represents the valence number of the substance's ions (electrons transferred per ion);
\(F\) – represents Faraday's constant (\(96,500 [C]\) or \(26.8 [Ah]\));
\(M\) – molar mass of the substance (material), in [g/mol].

Additionally, it can also be obtained from the electrochemical equivalence of typical materials that make up the element, and these are provided in tabular form; for example, Table 1 and Table 2 list several materials with their corresponding data.

The theoretical capacity of an electrochemical cell, based solely on the active materials participating in the electrochemical reaction, is calculated from the equivalent weight of the reactants. For example, the theoretical capacity of the \(Zn-Cl_2\) cell is \(0.394 [Ah/g]\), and it is obtained as follows:

For the reaction:

\[ Zn+Cl_2 \longrightarrow ZnCl_2\]

From Table 1 and Table 2, we extract the electrochemical equivalents of \(Zn\) and \(Cl_2\), yielding:

\[ 1.22 [g/Ah] + 1.32 [g/Ah] = 2.54 [g/Ah] \; or \; 0.393 [Ah/g] \]

or using the previous equation for the theoretical capacity, obtained from Faraday's Law:

\[ q = {{z \cdot F} \over {M}} = {{2 \cdot 26.8 [Ah][mol^{-1}]} \over {M(Zn)[g][mol^{-1}] + M(Cl_2) [g][mol^{-1}]}} = {{53.6[Ah]} \over {(65.4+71)[g]}} = {{53.6[Ah]} \over {136.4[g]}} = 0.393 {[Ah] \over [g]} \]

The theoretical values above were obtained only considering the material used for the anode and cathode, while the electrolyte or any other material was omitted. For example, in the case of the lead-acid battery, for the reaction:

\[ Pb+PbO_2+2H_2SO_4 \longrightarrow 2PbSO_4+2H_2O \]

If we also take into account the electrolyte, we will obtain the theoretical capacity of:

\[ q = {{z \cdot F} \over {(M(Pb)+M(PbO_2)+2M(H_2SO_4)}}={{2\cdot26,8[Ah][mol^{-1}]} \over {(207+239+2\cdot98) [g][mol^{-1}]}}={{53,6} \over {642}} {{[Ah]} \over {[g]}}=0,0835 {{[Ah]}\over{[g]}}=83,5 {{[Ah]} \over {[kg]}} \]

and this is much smaller than that in Table 4 where the authors² only considered the mass of the cathode and anode, thus obtaining \(120 [Ah/kg]\).

Theoretical energy

Considering the two quantities defined earlier, capacity and theoretical voltage, we can define the theoretical energy as being²:

\[ Watt \; hour [Wh] = Voltage [V] \cdot Ampere \; hour [Ah] \]

Taking the previous example of an element \(Zn-Cl_2\) for which a theoretical potential of \(2.12 [V]\) and a theoretical capacity of \(0.394 [Ah/g]\) were obtained, applying the previous equation we will obtain a theoretical specific energy:

\[ Specific_{energy}= 2.12 [V] \cdot 0.394 [Ah/g] = 0.835 [Wh/g]=835 [Wh/kg] \]

If we denote the specific energy by \(w\) and take into account the previous equations, we can write the theoretical energy equation as:

\[ w = q \cdot \Delta E^0 \]

Thus, in the case of the lead-acid battery, where we consider the voltage per element to be \(2 [V]\), we will obtain a specific energy of:

\[ w = q \cdot \Delta E^0 = 83.5 {{[Ah]} \over {[kg]}} \cdot 2[V] = 167 {[Wh] \over [kg]} \]

The values obtained in practice are much lower. The actual mass of the lead battery consists, in addition to the material that makes up the electrodes (anode and cathode), of: electrolyte, separators, casing, terminals, etc.

The maximum electrical power supplied by a battery/element is obtained when half of the nominal voltage⁶ is reached, in other words:

\[ P_m = {{1} \over {2}} \cdot I \cdot U_0 \]

, where:
\(P_m\) - represents the maximum power;
\(I\) - expresses the discharge current;
\(U_0\) - indicates the nominal voltage of the battery/element.

Charge-discharge rate – C – rates

To indicate the discharge rate, respectively the charging rate, the so-called expression C rate is used in English. This is determined using the equation²:

\[ I = M \cdot C_n \]

, where:
\(I\) – represents the discharge/charge current, in amperes \([A]\);
\(C\) – represents the declared capacity, in ampere-hours \([Ah]\);
\(n\) – represents the time base, in hours \([h]\), at which the capacity was declared;
\(M\) – multiple under fractions of \(C\).

For example, a battery declared as \(5 [Ah]\) at a discharge rate of \(5 [h]\) (\(C_5=5 [Ah]\)) will have a discharge rate for \(0.1C_5\) or \(C_5/10\) of \(0.5 [A]\).

The capacity of a battery at \(1C\) means that a fully charged battery at \(1 [Ah]\) must provide \(1 [A]\) for one hour, \(1 [h]\). The same battery that discharges at \(0.5C\) should provide \(0.5 [A]\) for two hours, \(2 [h]\), and at \(2C\) it will deliver a current of \(2 [A]\) for 30 minutes or half an hour, \(0.5 [h]\). Losses during rapid discharges reduce the discharge time and at the same time these losses will also affect the charging time.

Manufacturers rate the capacity at different rates \(C\). In general, for batteries used in traction, due to the specific working time of \(8 [h]\) for a work shift, they are rated at \(C_5\), while those for stationary applications are generally rated at \(C_{10}\) or most often at \(C_{20}\) for marketing reasons. Therefore, we need to pay attention to the battery capacity, in \([Ah]\), at what rate of \(C\) it is defined. In other words, a battery rated with a capacity of \(100 [Ah]\) at \(C_{10}\) has a greater capacity compared to a battery rated also at \(100 [Ah]\) but at \(C_{20}\).

The use of batteries

The electrical energy generated by the renewable energy system, in this case photovoltaic, needs to be stored in a storage battery system. This type of system is also known as an off grid system because the renewable energy generation system is not connected to the National Electric System (SEN). There are also on grid systems where the storage system is absent, and in these cases, the electric energy produced by the renewable energy generation system is delivered directly to the National Electric System (SEN).

The storage system using storage batteries is suitable for use when there is a need to supply electric energy to equipment, namely electrical devices in the following cases:

• The absence of an overhead power line (LEA) or an underground power line (LES) that is part of the electric energy distribution system and connected to the National Electric System (SEN), the case of the off grid system;
• Achieving a 'pure' continuous power supply free of 'noise', in the case of communication systems. In this case, the storage system can operate both powered by a renewable system and by the National Electric System;
• Achieving high currents, respectively high powers, for a very short time, in the case of electrical equipment or devices that require high currents and high powers for a very short time, is encountered in electric drives (electric forklifts, electric stackers, automobiles, etc.). Additionally, they are also used when it is not necessary to contract a large installed power to cover short-term load peaks, or in cases where it is not possible to contract a larger installed power from the electric distribution system operator, so that it is ultimately used for a very short period of time. The contracted installed power is paid for each hour over the entire duration of the contract; additionally, it is necessary to size the electrical installations according to the required installed power from the connection point indicated by the distribution system operator;
• In the implementation of backup systems necessary for powering electrical equipment that must be continuously supplied without interruption of electrical energy, such as life support equipment, signaling, etc.

The on grid system is used when the battery storage system is not critically necessary and obviously when we have an electrical line connected to a distribution system. This type of system for the production and use of electric energy has the significant advantage that it is no longer necessary to invest in the storage battery system, but it necessarily involves obtaining approval from the distribution/transport system operator as well as a joint operating program for the respective electric line. It is also necessary for the measuring device used at the connection point to be bidirectional, meaning it must be able to measure both the electric energy injected into the energy system and the energy consumed from the energy system, which can be connected to the National Electric System (SEN) or can be a closed electric energy distribution system.

Lead-acid storage batteries

Storage batteries are devices composed of one or more elements that allow the accumulation of electric energy in chemical energy, which can at any moment reconvert the accumulated chemical energy back into electric energy⁷.

Figure 3. Components of a lead-acid cell⁷

, where:
\(1\) – tubular bars of the positive grid, cast under pressure;
\(2\) – protective element of the positive grid with high resistance to acid and oxidation;
\(3\) – reinforcement of the positive tubes;
\(4\) – negative plate;
\(5\) – microporous separator with electrical insulating qualities;
\(6\) – lead alloy terminals cast in place;
\(7\) – screw cap or bayonet type;
\(8\) – acid-resistant tank and cap. The tank is transparent and graduated, while the cap is marked with the polarity of each terminal.

The main components of a lead-acid element

The positive electrodes (plates)

The differentiation of lead-acid batteries is achieved through the technology that underlies the construction of positive electrodes, which are made in the form of a grid and an active material.

Thus, we encounter the following types of positive plates/electrodes⁷:

• Planté type positive plates (electrodes):
  • The grid and the active material are one and the same;
  • The positive plate is made of pure lead;
  • The casting of the plate is done under pressure in a single piece;
  • On each side, there are numerous vertical strips to increase the surface area;
  • The active material consists of lead dioxide that forms on the surface of the vertical strips.
• Positive plane (electrode) plates:
  • The plate is in the form of a grid and is made of lead alloy;
  • The casting of the plate is done through gravitational casting;
  • The plate consists of a frame shaped as a square or rectangle made up of several horizontal and vertical bars (grid);
  • The active material is represented by a paste based on lead oxides deposited on the grid.
• Positive tubular (electrode) plates:
  • The grid is made of lead alloy;
  • The casting is done through pressure casting;
  • The plate consists of a number of tubes containing the active material, and the ends of the tubes are firmly attached using a polyethylene base;
  • The active material is represented by a powder of lead dioxide.

The electrodes (plates) are negative.

These are characterized by⁷:

• The shape is flat;
• The grid is made of lead alloy;
• The casting of the grid was performed through gravitational casting;
• The grid consists of a frame shaped as a square or rectangle formed by several horizontal and vertical bars;
• The active material is represented by a paste of lead dioxide that is deposited on the grid.

Active material

For the positive plate (electrode), we have lead dioxide, which is brown (chocolate-colored).
For the negative plate (electrode), we have spongy lead, which is gray (ash-colored).

The positive or negative beam

It is the association of plates with the same polarity⁷, where:

• Each plate has an end (tail);
• These ends of the plates are welded using a horizontal bar, which is in turn secured with a vertical bar to the positive or negative terminal.

Grouping (series connection)

It represents the association of two beams of different polarities, and the plates are isolated from each other using the separator⁷.

Separators

The main role of such a separator is to prevent short circuits. However, in addition to this primary objective, it must also fulfill many qualities and functions⁷:

• It must:
  • Avoid any short circuit made directly or indirectly between a positive plate and a negative one;
  • Allow the passage of ions;
  • Maintain the necessary distance between plates;
  • Allow the diffusion of the electrolyte;
  • Allow the release of gases;
  • Be chemically neutral;
  • Exhibit good resistance to oxidation and acid.

The main base materials used in the construction of these separators are:

• Paper or polyester impregnated with phenolic resin;
• PVC;
• Polyethylene;
• Rubber.

The electrolyte

The electrolyte is a solution of sulfuric acid \(H_2SO_4\), diluted with water. Its density varies depending on the type of accumulator and the location (geographical area) of use⁷.

Table 5. The density of the electrolyte depending on the geographical area and type of element (prod. OLDHAM)

Element type	Temperate climate \([g/cm^3\)]	Tropical climate \([g/cm^3]\)
Positive plates of the Planté type	1.210	1.200
Flat positive plates	1.250	1.240
Tubular positive plates	1.265	1.250

The tank (the container)

The tank is a container made from an insulating material that cannot be attacked by sulfuric acid and is made from ebonite, polypropylene (PP), Acrylonitrile Butadiene Styrene (ABS), etc. Its purpose is to maintain the group of positive and negative plates in the electrolyte⁷.

The cap and the plug

The cap is usually made of the same material as the container (tank). The cap, after the plates are inserted, is glued or welded to the tank, and the passage of the terminals through it is sealed with rubber grommets. The plug is removable, acid-resistant, and must allow the passage of gases but not of the electrolyte⁷.

Elementul

Represents the complete accumulator⁷. The electromotive force of an element is \(2.1 [V]\). By convention, the nominal voltage of an element is considered to be \(2 [V]\). There are monoblock accumulators made up of 3 elements, with a nominal voltage of \(6 [V]\), and of 6 elements, with a nominal voltage of \(12 [V]\).

The Battery

Represents the assembly of elements grouped in series/parallel, and the connection between elements is made through flexible or rigid bridges or connections⁷.

Operating Principle

Discharge represents the transformation of chemical energy into electrical energy. Positive and negative active materials are sulfated through the electrolyte (sulfuric acid solution). The density of the electrolyte decreases during discharge ⁷.

Charging represents the transformation of electrical energy into chemical energy. Active and positive materials are de-sulfated through the electrolyte. The density of the electrolyte increases during charging until it returns to nominal values.

The lead-acid battery uses lead dioxide, \(PbO_2\), as the active material of the positive electrode and pure lead, \(Pb\), in a porous structure, as the active material of the negative electrode, while for the electrolyte it uses a solution of sulfuric acid, \(H_2SO_4\), at a concentration of \(1.28 [g/cm3]\) diluted with water².

During the discharge of the element, both electrodes experience the phenomenon of sulfation, transforming into lead sulfate, \(PbSO_4\). The process is reversed during charging; the element is desulfated. The chemical equations are²:

At the negative electrode:

\[ Pb \rightleftharpoons Pb^{2+}+2e\]

\[ Pb^{2+}+SO_4^{2-} \rightleftharpoons PbSO_4 \]

resulting in:

\[ Pb+SO_4^{2-} \rightleftharpoons PbSO_4+2e \]

At the positive electrode:

\[ PbO_2+4H^++2e \rightleftharpoons Pb^{2+}+2H_2O \]

\[ Pb^{2+}+SO_4^{2-} \rightleftharpoons PbSO_4 \]

resulting in:

\[ PbO_2+4H^++SO_4^{2-}+2e \rightleftharpoons PbSO_4+2H_2O \]

The overall electrochemical reaction is the sum of the two equations resulting at each electrode:

\[ Pb+PbO_2+2H_2 SO_4 \rightleftharpoons 2PbSO_4+2H_2O \]

During charging, as the element approaches full charge and most of the lead sulfate, \(PbSO_4\), has been converted into lead, \(Pb\), or lead dioxide, \(PbO_2\), the cell voltage during charging becomes greater than the gassing voltage (approximately \(2.39 [V]\)) and overcharge reactions will occur, leading to the production of hydrogen and oxygen (gases) and resulting in a loss of water from the electrolyte².

The reactions, in the case of exceeding the charging voltage of \(2.39 [V]\) per element, are:

at the negative electrode:

\[ 2H^++2e \longrightarrow H_2\]

at the positive electrode:

\[ H_2 O-2e \longrightarrow {{1}/{2}} \; O_2+2H^+ \]

resulting in:

\[ H_2 O \longrightarrow H_2+1/2 \; O_2 \]

In sealed lead-acid cells, this reaction is controlled to minimize water loss by recombining the oxygen produced at the negative electrode. The cell is designed such that the produced oxygen, \(1/2 \; O_2\), diffuses to the negative electrode, from \(Pb\), where it will form lead oxide, \(PbO\), which will interact with sulfuric acid, \(H_2SO_4\), resulting in lead sulfate \(PbSO_4\) and water, \(H_2O\)².

Figure 4. The voltage and specific density of the lead-acid battery at a constant discharge/charge rate²

Modification of the volume of active materials

The porosity and volume of active materials change during chemical reactions. Porosity is maximum when the accumulator is charged. The presence of pores in the active materials is necessary⁷:

• So that the electrodes are permeable to the electrolyte;
• To enable the alternation of sulfation and desulfation without destroying the structure. For the positive electrode, the transformation of lead dioxide \(PbO_2\) into lead sulfate, \(PbSO_4\), is accompanied by a volume change of 83[%].

For the negative electrode, the transformation of spongy lead, \(Pb\), into lead sulfate, \(PbSO_4\), is accompanied by a volume change of 165[%].

Considering these large volume variations of the active materials, if the active materials were to be fully utilized, it would result in irreparable damage to the electrodes. For this purpose, the active mass is used only 30[%], and this is achieved by limiting the discharge to a voltage known as the cut-off voltage⁷.

Electromotive force – open-circuit voltage

Electromotive force or open-circuit voltage represents the algebraic difference between the potential of the positive plate and the potential of the negative plate. These voltages can be measured in the laboratory using an electrod of: cadmium or mercuric sulfate. The difference between these two potentials gives the electromotive force⁷.

Table 3 6. Table of voltages⁷

	Positive plate	Negative plate	Voltage on element
	[V]	[V]	[V]
In discharge	1.9	0.2	1.7
At rest	2.21	0.11	2.1
In floating (charging)	2.3	0.1	2.2
At the end of charging \(0.03C_{10}\)	2.4	-0.3	2.7

The nominal voltage of a lead-acid element is \(2[V]\), the open-circuit voltage being a direct function of the concentration of the electrolyte. Thus, it varies from \(2.125[V]\), for an element with an electrolyte having a specific density of \(1.28[g/cm^3]\), to \(2.05[V]\), for an element with a specific density of \(1.21[g/cm^3]\). The cutoff voltage of discharge, at a moderate rate, is \(1.75[V]\) per element, but it can drop to \(1[V]\) per element at an extremely high discharge rate and at low temperatures, which is preferably to be avoided².

The terminal voltage is defined as follows⁷:

• While charging, the voltage varies between \(2.1[V] – 2.8[V]\):

  \[ U=E+R \cdot I\]

• While discharging, the voltage varies between \(2.1[V] – 1.7[V]\):

\[ U=E-R \cdot I\]

, where:
\(U\) – represents the terminal voltage, in \([V]\);
\(E\) – represents the electromotive force, in \([V]\);
\(R\) – represents the internal resistance of the element, in \([Ω]\);
\(I\) – represents the current through the element, in \([A]\).

Self-discharge

It represents the natural phenomenon caused by oxidation and reduction reactions that occur spontaneously at both positive and negative electrodes⁷.

• At the positive electrode:

  \[ PbO_2+H_2SO_4 \longrightarrow PbSO_4+H_2 O +1/2 \; O_2 \]

• At the negative electrode:

\[ Pb+H_2SO_4 \longrightarrow PbSO_4+H_2 \]

\[ Pb+1/2\;O_2+H_2SO_4 \longrightarrow PbSO_4+H_2O \]

Self-discharge can have high values as a result of other factors, such as internal reactions at the level of active materials where a phenomenon of micropiles occurs, due to the potential difference with the active lead of the plates and metallic impurities⁷:

• Present in the base materials;
• Resulting from the use of unsuitable or imperfectly demineralized/distilled water;
• Resulting from the natural or accelerated aging of the battery.

In general, self-discharge will depend on⁷:

• Electrochemical couple;
• The nature of the materials used in construction;
• Due to the design (concept used);
• Ambient temperature;
• Load during use;
• The number of charge and discharge cycles;
• Maintenance.

Figure 5. Self-discharge - Temperature Influence - BSB Solar 12-200⁸

Floating voltage – maintenance voltage

It represents the determined value of the voltage such that the current flowing into the battery is slightly in excess compared to the internal consumption current of the battery due to self-discharge⁷.

All lead-acid batteries, regardless of manufacturer, construction principle, or the nature of the materials used, have the same floating voltage.

Table 7. Floating voltages per element⁷

Open lead	2.20 [V]	Temp.: \(20 – 25^0C\)
Sealed lead	2.25 [V]	Temp.: \(20 – 25^0C\)

Considering that the terminal voltage depends on the temperature, a correction is made for temperatures above \(25[^0C]\) and below \(20[^0C]\)⁷:

• For temperatures below \(20[^0C]\), the necessary correction is \(+3[mV/K]\);
• For temperatures above \(25[^0C]\), the necessary correction is \(-3[mV/K]\);

As we have seen, an element is in floating when it is in the charging process and the voltage slightly exceeds the open-circuit voltage so as to cover the losses due to discharge. The value of the floating current will depend on the technology, but especially on the temperature and the density of the electrolyte, as the concentration of sulfuric acid, \(H_2SO_4\), increases the voltage of the two electrodes, the positive and the negative².

Thus, the electromotive force, with open terminals, varies depending on the concentration of acid. An increase in the concentration of sulfuric acid, \(H_2SO_4\), from 6[%] to 16[%] will increase the potential of the positive electrode by \(0.06[V]\) and that of the negative electrode by \(0.004[V]\), representing an increase per element of \(0.064[V]\)⁷.

Table 8. The electromotive force (voltage) as a function of the concentration of sulfuric acid⁷

Density at \(25^0C\)	Concentration of the acid, \(H_2SO_4\)	Electromotive force
\([g/cm^3]\)	[%]	\([V]\)
1.040	6.04	1.89
1.100	14.72	1.96
1.150	21.38	2.00
1.200	27.68	2.05
1.250	33.80	2.10
1.280	37.40	2.13
1.300	39.70	2.15

Charging and discharging lead-acid batteries

A proper charge will result in extending the life of any lead-acid battery. To achieve this goal for lead-acid batteries during their charging, the following three rules must be observed⁷:

• The charging current, at the beginning of the charging process, can have any value that does not produce an increase in the voltage across the element greater than the gas evolution voltage (approximately \(2.4[V]\));
• Throughout the entire charging duration and until the completion of the recharge, the charging current will be monitored and controlled so that the voltage across the element remains lower than the gas evolution voltage. For a rapid charge, a current intensity just below this gas evolution voltage can be used. A voltage of approximately \(2.35[V]\) per element is considered safe;
• When the recharging is completed, the battery has regained very close to 100[%] of its nominal capacity, the charging current will be completed and limited to \(C_{20}\) (for example, \(10[A]\) for a battery with a capacity of \(200[Ah]\)). After completion, the charging current will be reduced so that the voltage on the element equals the floating voltage (maintenance voltage).

The principle of charging

During charging, the following phenomena occur⁷:

• The electrodes will regenerate sulfuric acid through desulfation;
• The electromotive force will increase;
• The internal resistance will decrease as a result of the increase in concentration in the electrodes;
• When the voltage across the element reaches \(2.35[V]\), gas will begin to appear at those two electrodes;
• The voltage of the negative electrode will change sign at the end of charging so that it will add to the positive voltage. This change in direction causes the voltage across the element to increase sharply;
• The fact that the voltage at the terminals, the density of the electrolyte will become nominal and constant, and gas emissions will occur are indications that the end of charging has been reached;
• Continuing the charging will become an overload for the battery and will cause the entire current to be used for the decomposition of water into hydrogen and oxygen.

Charging at constant current

Due to the fact that charging involves current control, this type of charging is not used for charging lead-acid batteries. This method is only used when small currents are employed for charging, obviously resulting in a significant increase in charging duration to 12 hours or more. The current intensity is limited to \(0.03C_{10}\) and charging is interrupted when the temperature on the element reaches \(40[^0C]\)^{2 7}.

Figure 6. Charging at constant current²

Charging at constant voltage

This charging method is the most commonly used method for both stationary lead-acid batteries and traction lead-acid batteries. The characteristics of the current and voltage curves during constant voltage charging are presented in the figure below:

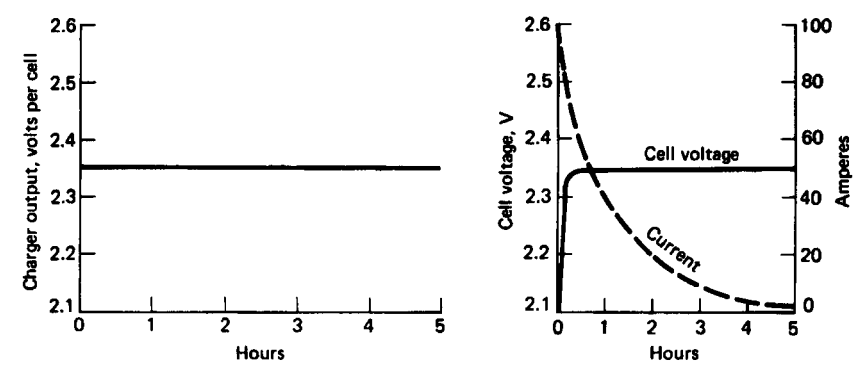
Figure 7. Constant voltage charging²

In this case, the charging circuit also features a current limiting system restricted to \(0.1C_{10}\)².

The constant charging voltage represents a voltage higher than the floating voltage, ranging between \(2.25[V]\) and \(2.35[V]\) per element. Additionally, in this case, we will also aim not to exceed the gas evolution voltage of approximately \(2.4[V]\) per element, so the charging device will have a voltage limit of \(2.39[V]\) or will automatically disconnect upon reaching this voltage on the element^{2 7}.

Pulse charging

This type of charging is used for lead-acid batteries employed in traction as well as for stationary ones used in conjunction with solar panels. In the case of this type of charging, the charger is disconnected from the battery for a period during which the open-circuit voltage across the battery/element is measured. Considering this aspect, the disconnection of the source from the load, this type of charging is not used in the case of wind generators where it is prohibited to let the wind generator run idle².

Principally, this type of charging stops when the measured open-circuit voltage per element exceeds a certain set threshold.

Slow charging (trickle charging)

A slow charge is essentially a constant current charge, with a low rate of approximately \(C/100\), which is used to keep the battery in a charged state. It is also used for restoring capacity when batteries are used intermittently².

Fast charging

In some applications, it is necessary to restore the nominal capacity in a very short time (one hour or less), which is usually encountered with lead-acid batteries used for traction (for example, in submarines). To avoid irreparable damage to the battery, the charging circuit closely monitors: temperature and voltage on the element and will thus control the recharge rate and charging speed, to the maximum possible, by limiting overcharging and the occurrence of gases².

Discharge

During discharge, the density of the electrolyte will progressively decrease due to the diffusion of acid to the electrodes, which will sulfate, and the internal resistance will increase. The variation in voltage across the element is approximately 0.3[V], and the electromotive force will decrease more rapidly as the discharge current increases⁷.

Thus, if we look at the catalog sheet of the battery BSB Solar 12-200, we will find that the same battery is marked at different rates \(C\) depending on the discharge current and also based on the depth of discharge (DOD – Depth of Discharge), which will affect the number of possible recharge cycles⁸.

Table 9. The capacity of a BSB Solar 12-200 battery as a function of the discharge current⁸

Time [h]	1	3	5	10	24	48	72	100	120
Final voltage [V]	1.8	1.8	1.8	1.8	1.8	1.8	1.8	1.8	1.8
Capacity [Ah]	130	150	171	200	214	224	233	240	243

As we can see from the table, the same battery BSB 12-200 that is marked and sold by the manufacturer at \(C_{10}\) as having a capacity of \(200[Ah]\) will have a capacity at \(1C\) of only \(130[Ah]\), while at \(C_{120}\) its capacity will increase to \(243[Ah]\).

Figure 8. Number of possible cycles vs DOD for the BSB Solar 12-200 battery⁸

From the figure above, we observe that in the case of a battery, its lifespan, which translates to the possible number of charge-discharge cycles, decreases dramatically with the increase in depth of discharge (the extraction of a large amount of energy from the battery). Thus, in the case of discharges of 100[%], our battery will have only 300 cycles, while in the case of discharges of approximately 20[%], the number of cycles will increase significantly to 2400 cycles. This will be taken into account in the design of a lead-acid battery system, and a DOD percentage of 70[%]-80[%] will be considered.

A battery is considered to have reached the end of its life when its capacity has dropped to 80[%] of its initial capacity after a full charge cycle⁹.

Figure 9. Main features of the BSB Solar 12-200 battery

The main features, as presented in the catalog sheet, of the BSB Solar 12-200 battery are shown in the figure above.

From this, we can find out that:

• Model name Solar 12-200;
• The battery life maintained in floating mode – fully charged and where losses due to self-discharge are recovered with a charger – has a duration of 15[years];
• The operating duration in operating mode – 5[years] – which represents for a battery \(C_{10}\) at a full cycle at \(24[h]\) approximately \(1800\) cycles. From which it follows that we will obtain these characteristics for a DOD of approximately 70[%];
• The capacity at \(20[^0C]\), for different discharge rates \(C\);
• Dimensions of \(522[mm]x238[mm]x218[mm]\);
• Weight, of \(64[kg]\);
• Internal resistance when it is charged, at \(20[^0C]\), \(3[mΩ]\);
• Self-discharge, in [%], for each month, at \(20[^0C]\), of 3[%/month];
• Capacity at \(C_{10}\) affected by temperature;
• Charging voltage, in \([V]\), for operating cycles \(14.4[V]-14.8[V]\) at a maximum current of \(50[A]\) and in floating of \(13.6[V]-13.8[V]\).

The maximum current is the short-circuit current per element, determined using the internal resistance:

\[ I_{sc} = {{U} \over {R}} ={{2.1}\over {({0.003}/6)}} {{[V]} \over {[\Omega]}} = 4200 [A] \]

, where:
\(I_{sc}\) – represents the short-circuit current, in \([A]\);
\(U\) – represents the voltage across the element, in \([V]\);
\(R\) – the internal resistance of an element, in \([Ω]\).

Battery sizing

The sizing of a battery system is done taking into account the characteristics of the consumer⁷.

Sizing under constant current conditions

The design data are:

Table 10 Known data for design

Power - P	2.5	\([kW]\)
Minimum voltage - Umin	43	\([V]\)
Maximum voltage - Umax	54	\([V]\)
Autonomy	10.0	\([h]\)
Temperature	20.0	\([^0C]\)

The determination of the maximum number of elements required will be made considering the maximum voltage needed and the voltage per element when it is charged⁷:

\[ N_{el} = {{U_{max}} \over U_{el_{inc}}} = {{54} \over {2.25}} = 24 \]

The minimum voltage per element is determined for the most unfavorable case when the battery has reached the minimum voltage relative to the number of elements determined previously⁷:

\[ U_{el(min)} = {U_{min} \over N_{el}} = {{43}\;[V] \over {24}} \cong 1.8 [V] \]

The determination of the maximum current that will be requested from the battery will be made considering the most unfavorable case we encounter when the voltage is at a minimum, so that we can ensure the nominal power of the load⁷:

\[ I_{desc} = {{P} \over {U_{min}}} = {{2500} \over {43}} {{[W]} \over {[V]}} = 58.14 [A] \]

From the resulting data, we will need to create a battery made up of \(24\) elements that must provide \(58.14 [A]\) for \(10 [h]\), and at the end of these hours, the voltage per element should not be less than \(1.8 [V]\).

With the previous data and from the analysis of the offers, I settled on the OPzV2-600¹⁰ element, which provides a current of \(58.8 [A]\) for \(10 [h]\), and the voltage at the end of these hours is \(1.83 [V]\). Such an element would have a quite significant cost.

In cases where we have consumers that also have short-duration current peaks, our battery will be supplemented, preferably with CapBat batteries (capacitor batteries) because they offer the advantage of numerous cycles, even with complete discharges, and the fact that they are capable of delivering a large amount of current in a very short time, as in this case, energy is stored directly in electric charges.